Main Index Page

Last updated 2/4/2024

x

This design appears to have been known at an early date in both Western Europe and Japan.

In Japan pentagonal knots have also been used from an early date, possibly an earlier date, to fasten articles of clothing.

There is a separate page about Japanese Knotted Letters, the knots in which are sometimes pentagonal and sometimes not.

There is also a separate page about Abd-El-Krim, a novelty figure made by combining two pentagonal knots.

**********

In Japan (and in books by Japanese authors)

1723

This print, also by Nishikawa Sukenobu, from 'Joro shina sadame', published in 1723, shows a pentagonal knot used to fasten the ties of a garment. The women in this print are members of the royal family.

**********

c1700 - 1750

This lacquered wooden box with a lid in the form of a pentagonal knot is held in the collection of the Victoria and Albert Museum in London.

**********

1758

This print from volume 1 of 'Ehon mitsuwa gusa', which was published in 1758, shows a document folded into a pentagonal knot laid in a box, possibly serving as a wrapper for some dried abalone.

**********

1768

In this print from 'Ehon ike no kawazu', which was published in 1768, the lady on the right is offering the man in front of her a stick to which a document is tied using a pentagonal knot.

**********

1899

In this print by Mizuno Toshikata from the picturebook 'Imayo bijin' which was published in 1899 the squatting woman's hair is secured using a pentagonal knot.

**********

1903

The Pentagonal Knot is pictured in 'Jinjo Kouto Shogaku Shuko Seisakuzu' (Handicrafts for ordinary higher elementary schools) by Hideyoshi Okayama was published by Rokushiro Uehara in Tokyo in 1903.

**********

1907

A drawing of the Pentagonal Knot appears in 'Shukou Tebikigusa : Kokumin Kyoiku Origami Yuihimo' by Ishin Nishigaki, which was published by Meguro Shoten in Nagaoka in 1907.

**********

1935

'Origami Moyo, Book Two' by Kawarazaki Kodo, which was published in Japan in 1935, contains a print showing a Pentagonal Knot.

**********

In Western Europe and the Americas

1668

The article shown below was printed on page 11 of issue 272 of the French journal 'Les Feuilles Marcophiles' in 1993. It shows two notes or letters which have first been folded into narrow strips and then into pentagonal knots and addressed on the outside, presumably for delivery by hand. The unfolded verso and recto of the left hand knot are also shown. Neither document bears a date. The article states that the assigned date of 1668 has been determined from other documents in the same archive. The name of the archive, however, is not given. My thanks to Michel Grand for this information.

**********

1682

A description of how to fold a pentagonal knot appears in the 1682 second edition of 'Trattato Della Sfera' in a section called 'Prattische Astronomonische. Intorno all circoli della Sfera' which was an addition to the original work by Urban d'Aviso and did not appear in the 1656 first edition. (The second paragraph explains how to fold a hexagonal knot.)

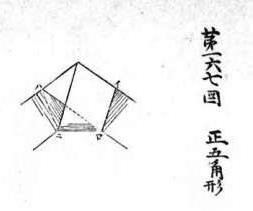
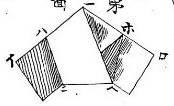
The first paragraph is translated in John Sharp's article 'Folding the Regular Pentagon' (BSHM Bulletin: Journal of the British Society for the History of Mathematics,31:3,179-188, 2016) as 'On the subject of drawing these figures, I want to give a way of describing, and forming mechanically, a Pentagon, which is one of the most difficult figures to draw, nevertheless it is the easiest, since it is found in nature ... because it is none other than a simple knot. You would take, for instance, a strip of paper, of whatever thickness you want, and which has two parallel sides, and with this proceed to make a knot, as if the paper were a cord, being attentive, however, that, first the paper is always in the same folds, and, second, that it is tightened sufficiently to remain well stretched. If you were now to cut the ends which stick out, with some scissors, you would have a most true pentagon.'

**********

1891

The Pentagonal Knot was published in L'Illustration 2527 of 1st August 1891 and subsequently included in the second volume of 'La Science Amusante' by Tom Tit (real name Arthur Good), which was published in Paris by Librairie Larousse in 1892.

**********

1892

The same design also occurs in 'Le Travail Manuel a L'ecole Primaire' by Jully & Rocheron, which was published by Librairie Classique Eugene Belin in Paris in 1892.

**********

1893

The Ring of Five Pentagonal Knots was published in L'Illustration 2613 of 26th March 1893 and subsequently included in the third volume of 'La Science Amusante' by Tom Tit (real name Arthur Good), which was published in Paris by Librairie Larousse in 1893.

**********

1904

The Pentagonal Knot appears in 'Guia Practica del Trabajo Manual Educativo' by Ezequiel Solana, which was published by Editorial Magisterio Español in Madrid in 1904, as a way of constructing a regular pentagon.

**********

1914

As 'El Pentagono' in 'El Trabajo Manual en la Escuela' by Félix Martí Alpera, which was published by Libreria de los Sucesores de Hernando in Madrid in 1914.

**********

1918

In 'Scientific Amusements' (an English translation of some of the material from volumes 2 and 3 of 'La Science Amusante' by Tom Tit) which was published by Thomas Nelson and Sons Ltd in London in 1918.

***

The Seven Pentagons

**********

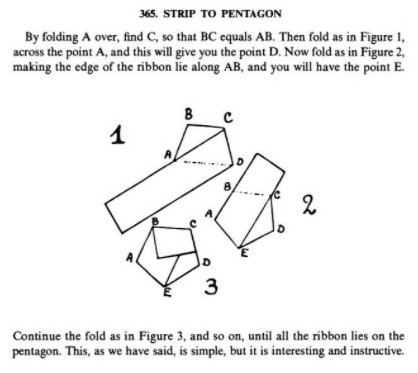
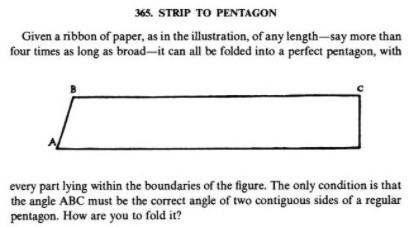
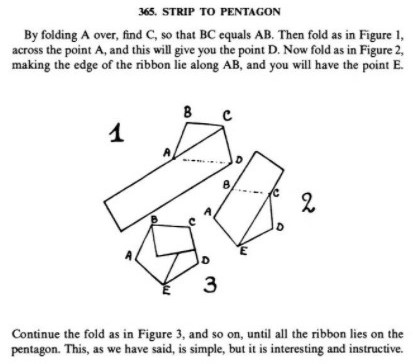
1926

As a puzzle in 'Modern Puzzles' by Henry Ernest Dudeney, which was published by C. Arthur Pearson in London in 1926.

Solution

**********

1928

On 26th June 1928 Miguel de Unamuno wrote a poem which refers to the pentagonal knot / pentagram:

'Dios jugando con los dobles / cinco dedos de ambas manos / anudó cinta de yerba; / de cinco puntas fue el lazo. / De donde sacó la estrella / pentagonal, que sus brazos / dio a la blancas frescas alas / de la rosa del garbanzo.'

In English, roughly:

'God playing with the doubles / five fingers of both hands / knotted weed tape; / Five-pointed was the loop. / Where did he get the star / pentagonal, than his arms / He gave the fresh white wings / of the garbanzo rose.'

This poem was included in 'Cancionero' (Songbook), a collection of Don Miguel's poetry published posthumously in 1953.

**********

1931

As a puzzle in 'Puzzles and Curious Problems', which was published by Thomas Nelson in London in 1931.

**********

1932

'Winter Nights Entertainments' by R M Abraham, which was first published by Constable and Constable in London in 1932, contains diagrams for the Pentagonal Knot under the title 'Here Is Another Method'. This title refers to the previous design in the book which was introduced with the words 'Here is one of the ways our grandmothers folded their love-letters ...'

**********

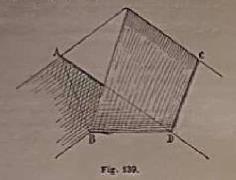
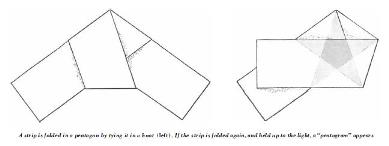
1933

R M Abraham's follow up book, 'Diversions and Pastimes', which was first published by Constable and Constable in London in 1933, included the See-Through Pentagram under the title of 'Five-Pointed Star'.

**********

1939

In 'Fun with Paper' by Joseph Leeming, which was published by Spencer Press Inc in Chicago in 1939.

**********

1940

'El Plegado y Cartonaje en la Escuela Primaria' by Antonio M Luchia and Corina Luciani de Luchia, which was published by Editorial Kapelusz in Buenos Aires in 1940 as a way of constructing a regular pentagon.

**********

1959

Both the Pentagonal Knot and the See-Through Pentagram were explained in an article titled 'About origami, the Japanese art of folding objects out of paper' by Martin Gardner which appeared in the July 1959 issue of 'Scientific American'.

**********

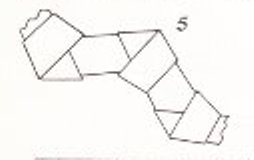
1960

'Paper Folding Fun' by Robert Harbin, which was published by Oldbourne in London in 1960,contains an illustration of a strip of Pentagonal Knots.

**********